Home Content Areas Math
Category:

Math

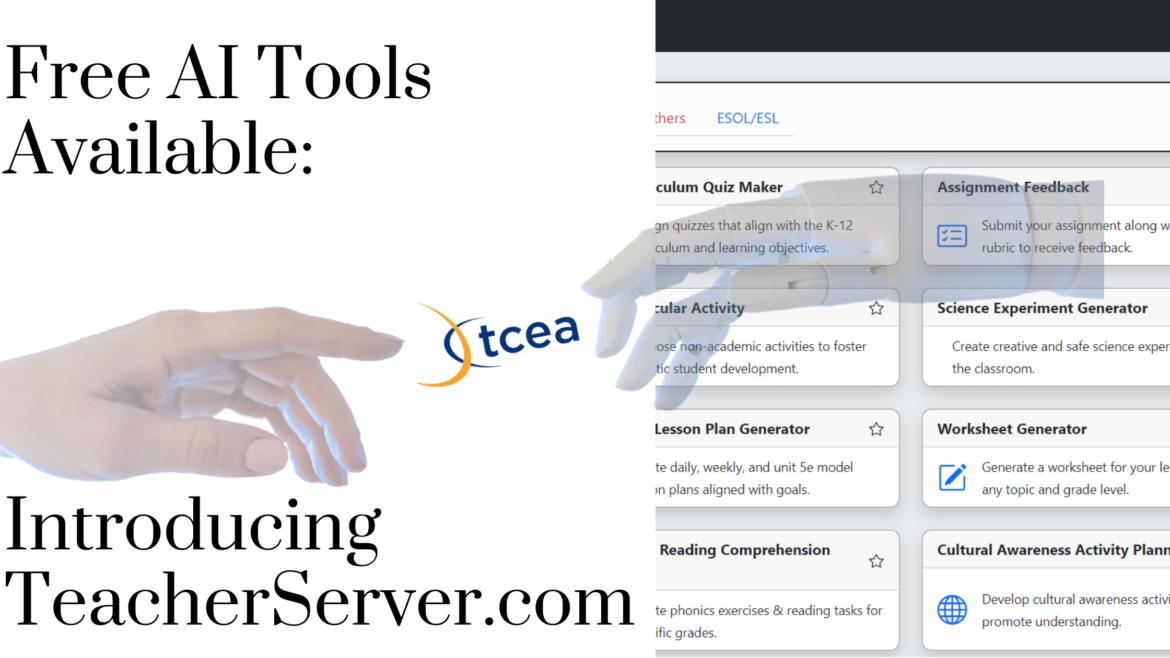
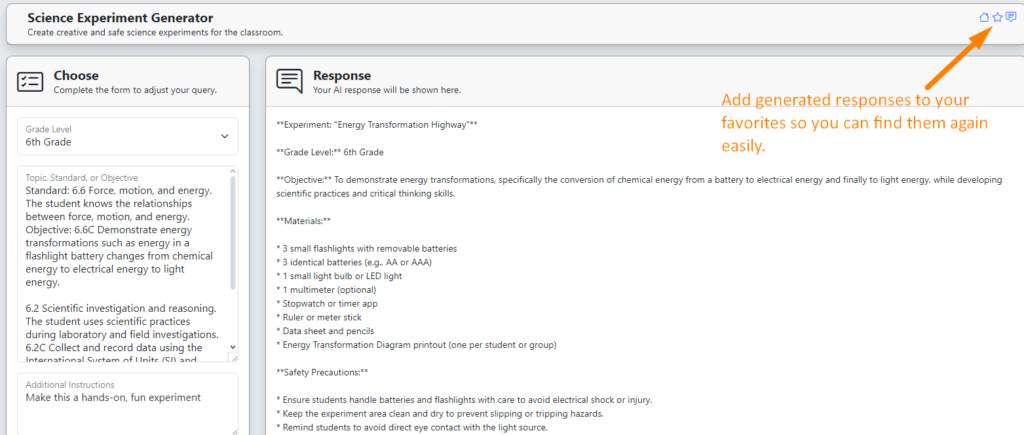
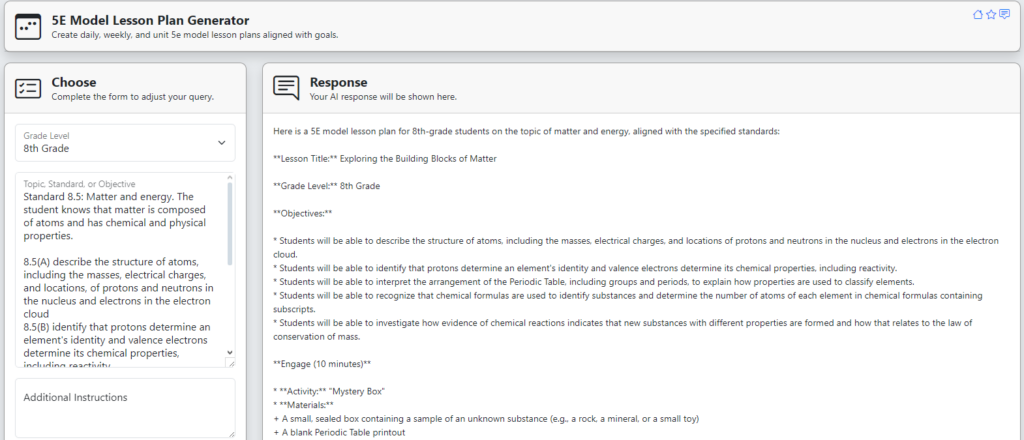
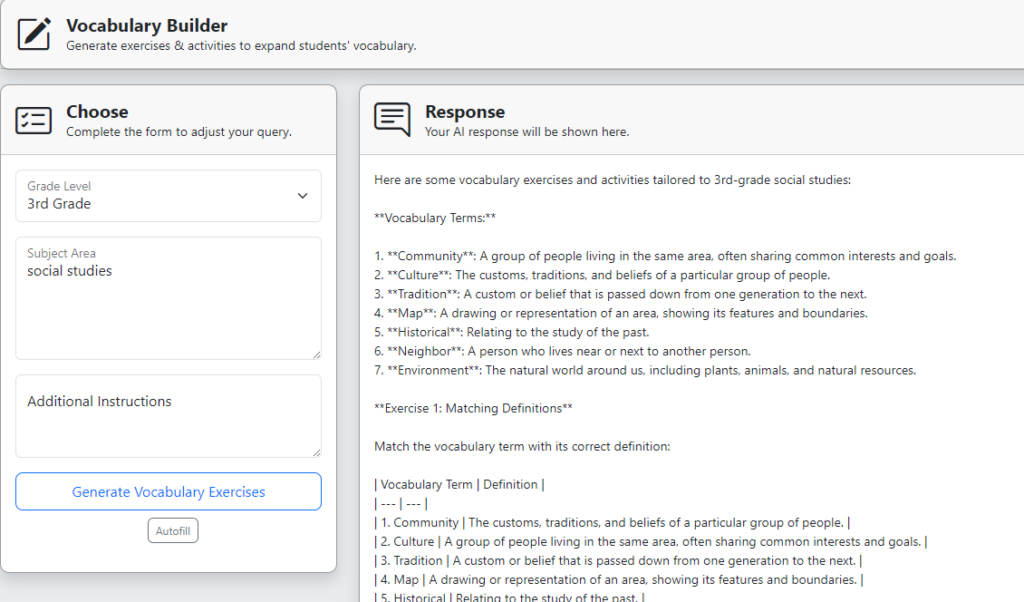
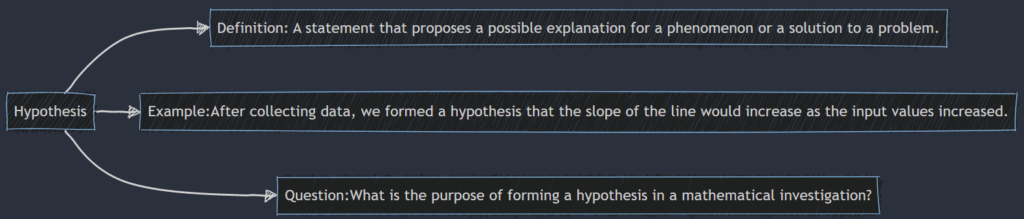
Discover resources, tools, and strategies for teaching math. Engage students with activities that build problem-solving and critical-thinking skills.

Tackle Word Problems in STRIDE:
Math

Tackle Word Problems in STRIDE

by Miguel Guhlin
written by Miguel Guhlin

“Math is my worst subject,” I often thought growing up. Never was this fact more evident than when my teacher presented me with a word problem. My mother, a veteran middle school math teacher, worked math problems and read Algebra textbooks the way I do books on writing. But the calculus of word problems continued to escape me even into college.

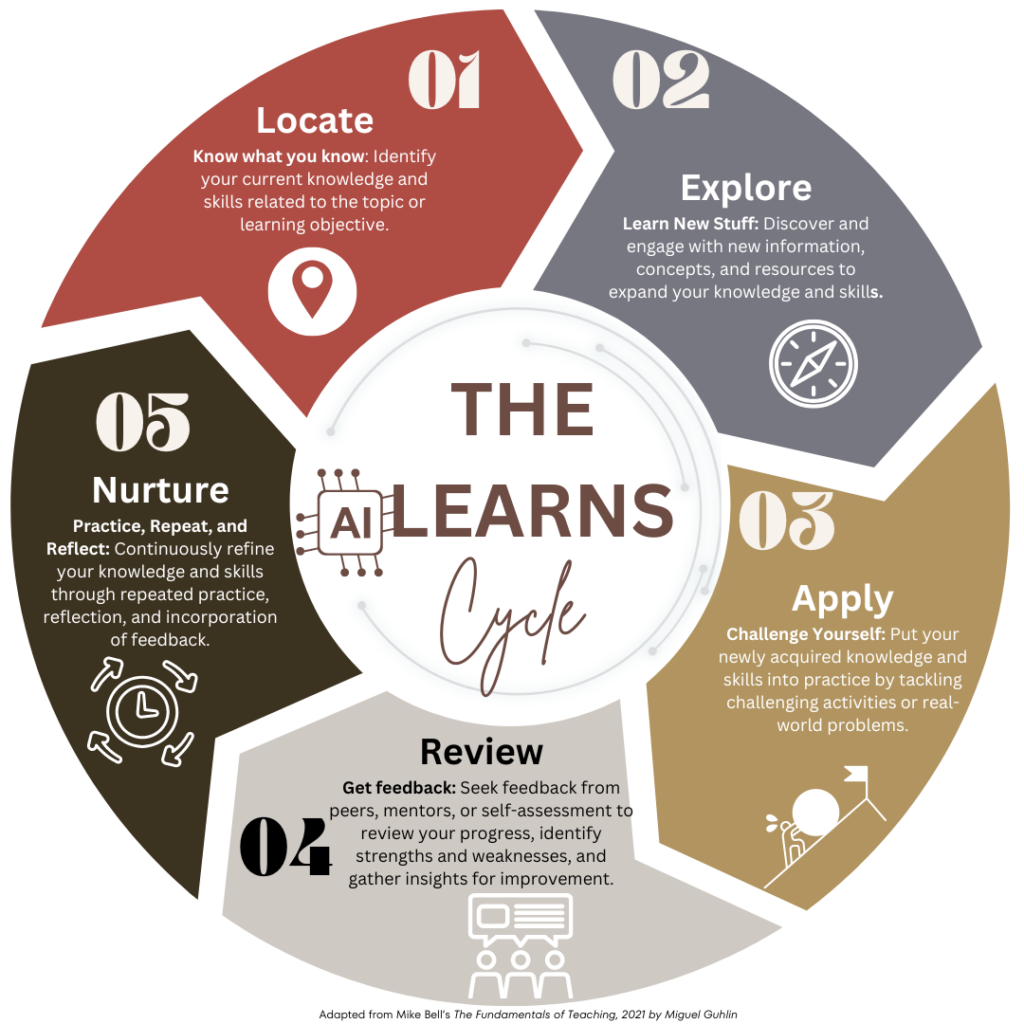
It’s no surprise that word problems intimidate. Having a framework to build problem-solving mastery may make the difference for students like me. I often wished for a simple approach to solving math problems, but my mother, who taught math for many years successfully, never gave me one approach or model. I always wonder, if we offered students one model or approach to use consistently, would that would work? Perhaps like George Polya’s approach or the STRIDE Model, which I will focus on in this blog.

Update: Lynn has made a compelling argument for modifying the STRIDE Model in the comments. I’ve responded with a revised model that seeks to capture the critique in her remarks. I’ve also included the revised version with a new infographic at the end of this blog post. You decide which is the better model, version 1 or version 2. My thanks to my readers for assisting me in learning how to solve math word problems, to leverage comprehension while solving them.

The STRIDE Model v1

The STRIDE Model (Scan, Think, Reflect, Identify, Do, Evaluate) equips learners with systematic steps. It facilitates breaking down problems into steps. The Model aligns itself to schema-based instruction, and critical thinking. Let’s explore how to integrate STRIDE into classrooms with grade-specific activities, templates, and actionable infographics.

Let me know what you think, non-math experts and fellow bewildered word problem-solvers. Does STRIDE work for you?

Image created by author

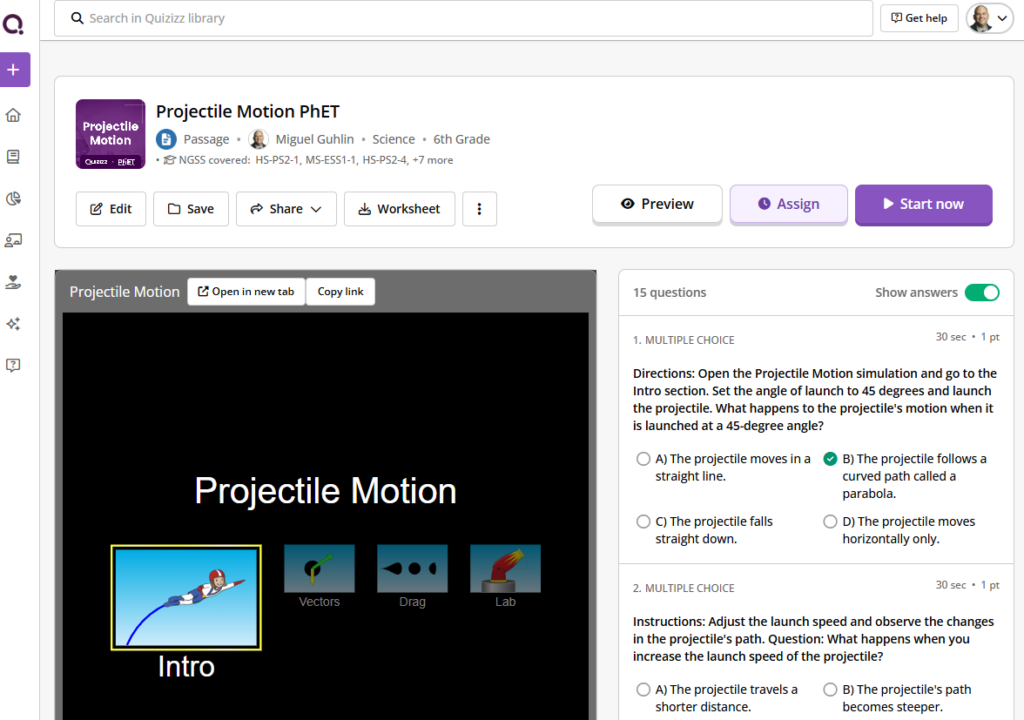
An Example Solution: The STRIDE Framework

Let’s explore what this might look like below. If you’re a math teacher, I hope you’ll double-check the math and share your thoughts in the comments. Be kind, I’m not a math person and I totally relied on AI for support.

Image created by author

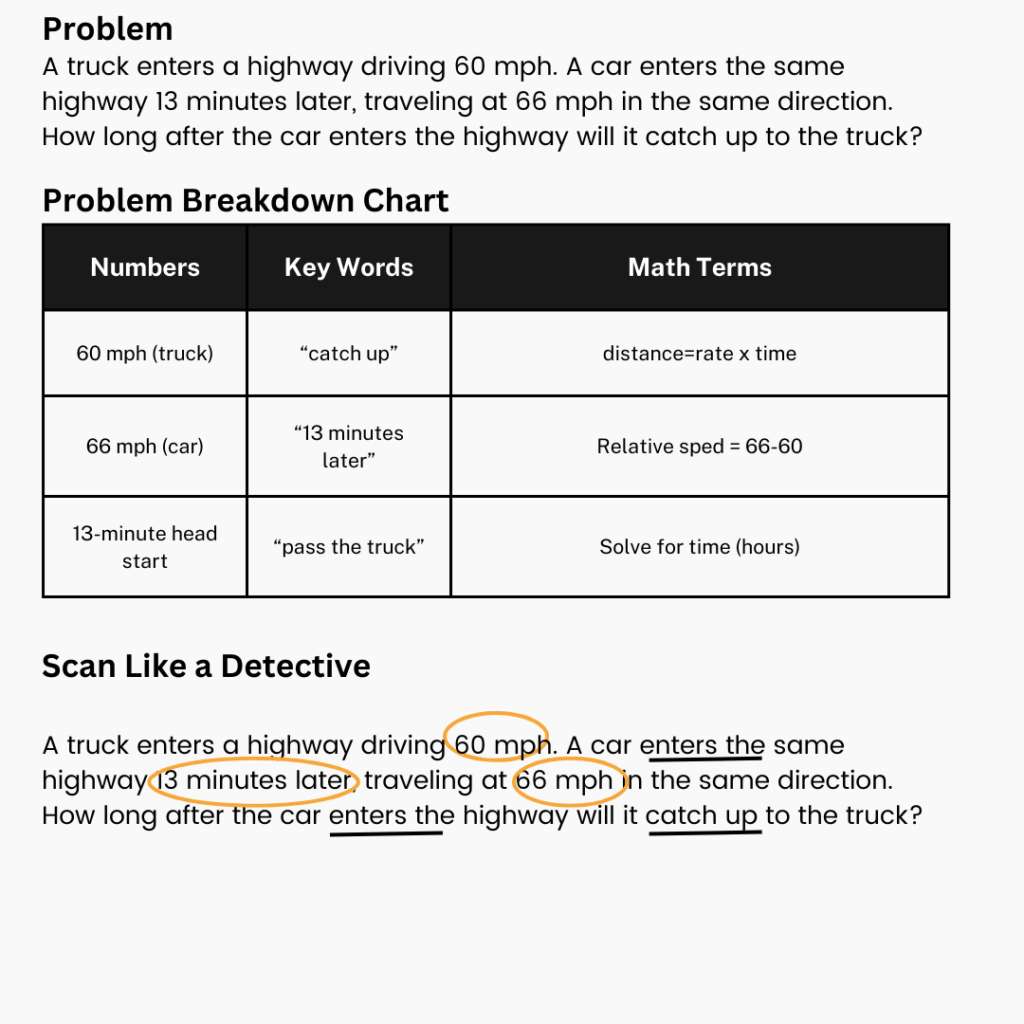
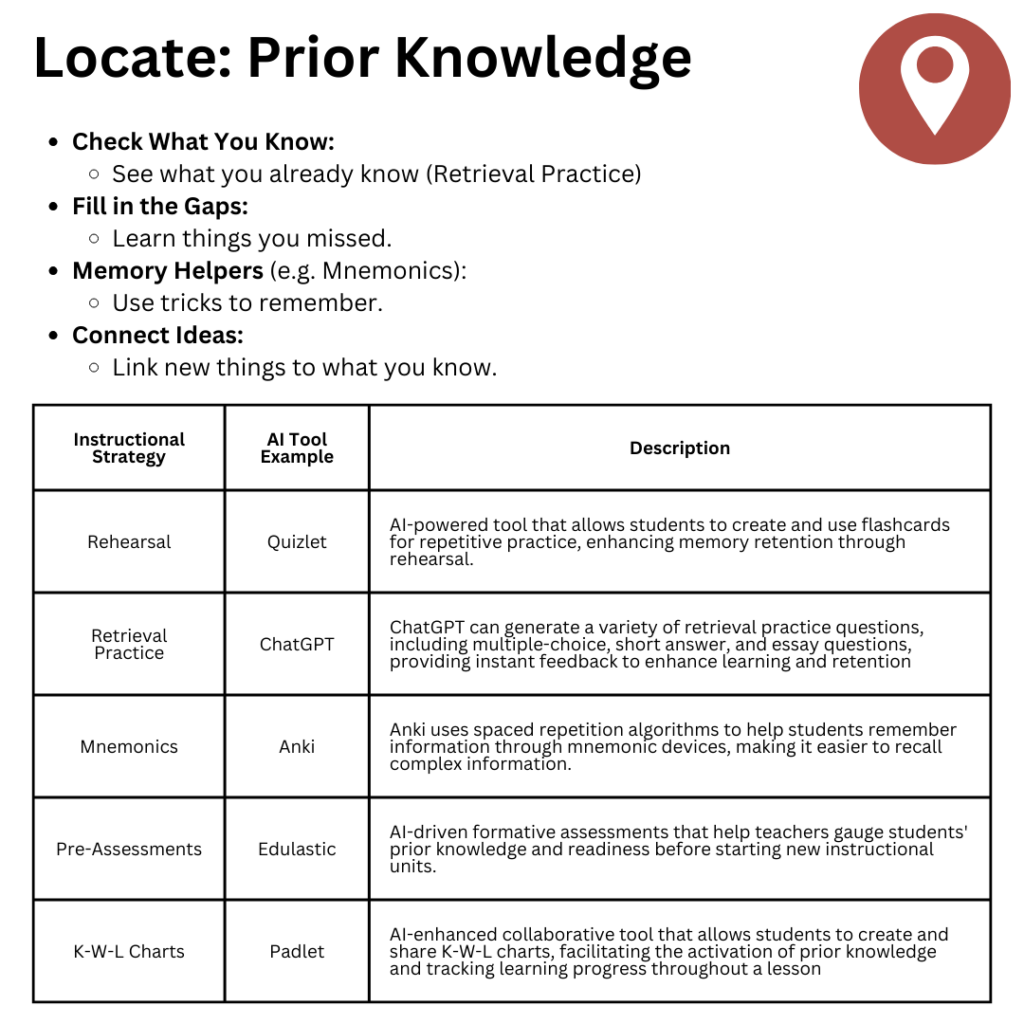
1. Scan the Problem

  • Numbers:
    • Truck speed = 60 mph,
    • Car speed = 66 mph,
    • Head start = 13 minutes.
  • Key Words: “13 minutes later,” “pass the truck.”

2. Think About Connections

  • Relate to real-life highway overtaking.
  • Visualize the truck’s head start as a distance gap.

3. Reflect on the Question

  • Rephrase: “How long will the car take to close the 13-minute distance gap created by the truck?”

4. Identify a Strategy

Calculate the truck’s head start distance:

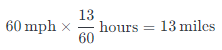
Determine the car’s relative speed:

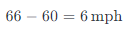
5. Do the Work

Time to catch up:

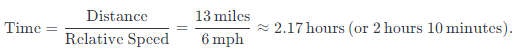
6. Evaluate Your Answer
Verify both of these items:
a) Truck’s distance after 2.17 hours:

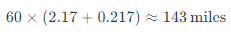
b) Car’s distance:

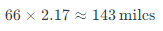
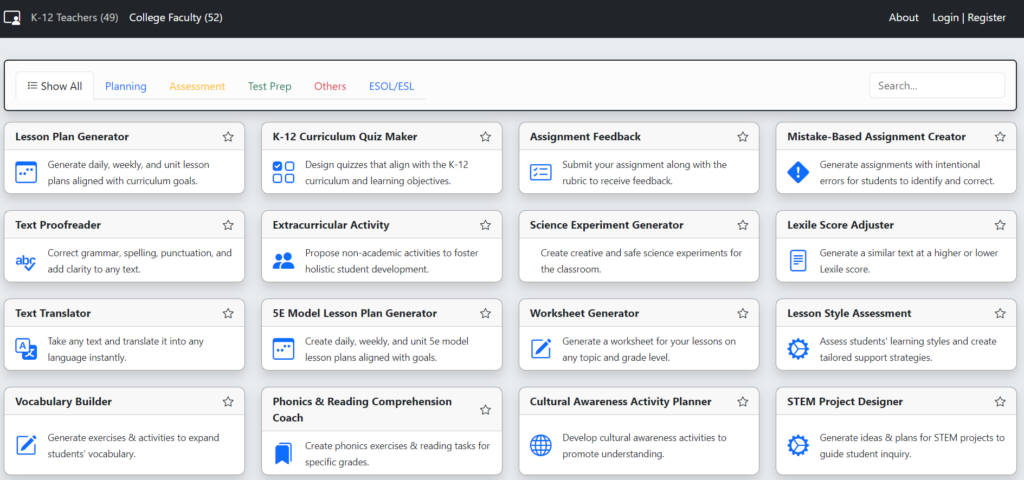
Digital Templates

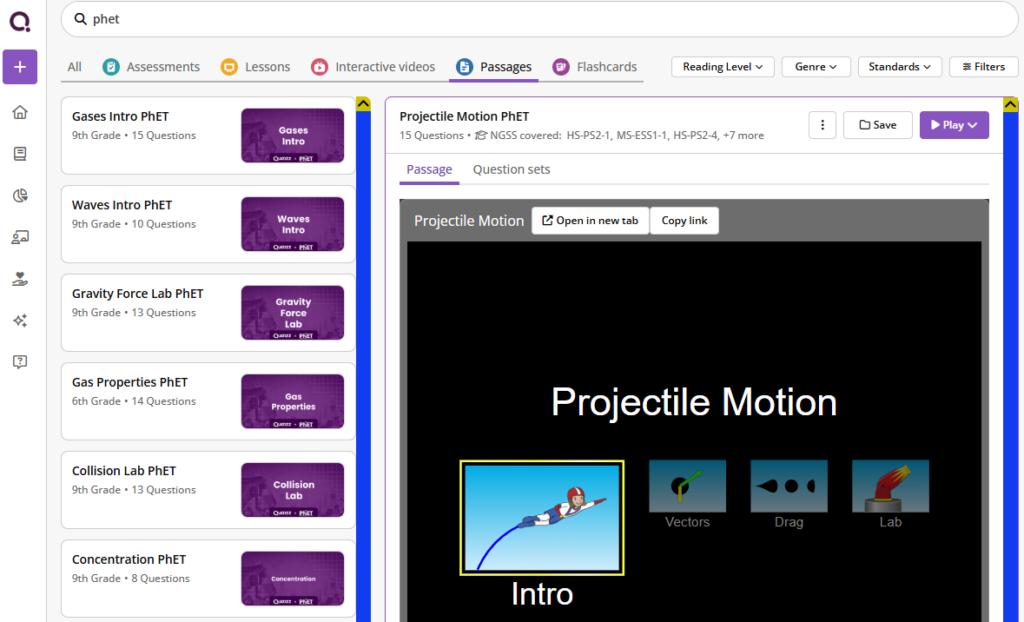
Below, you will find a chart that clarifies each stage of the STRIDE strategy, along with some sample ideas for activities. You can find a Canva template link to the STRIDE Model here.

StrategyActivitiesFocus
Scan the ProblemProblem dissection worksheets; keyword highlighting in multi-step problemsCircle numbers, underline keywords
Think About ConnectionsDrawing diagrams; writing real-life scenarios to contextualize problemsReal-life connections, diagrams
Reflect on the QuestionRephrasing exercises (individual worksheets and group comparisons)Goal identification, self-check prompts
Identify a StrategyStrategy justification; challenge prediction during planningBrainstorming, step-by-step planning
Do the WorkStep-by-step demonstrations (interactive whiteboards); collaborative solvingShowing work, explaining logic

Give it a go! Try students out on the STRIDE Model, a schema-based framework for solving word problems. You never know, it may assist them as they work to solve problems.

STRIDE Model v2

As promised, here’s the revised model. What do you think? How could it be improved based on your knowledge and experiences?

S – Survey the Situation:

  • Read the entire problem carefully to understand the overall story or context. What is happening in the problem?
  • Identify all the important information given: What facts or details do you know? (Make sure to include numbers and their units or labels).
  • Briefly consider what the problem might be asking you to find or do (you’ll refine this in step R).

T – Think About Connections:

  • Connect the problem to what you already know (similar problems, real-world experiences).
  • Visualize or draw the situation described in the problem to help build understanding and see relationships between the pieces of information.

R – Reflect on the Question:

  • Ask yourself: What specifically is the problem asking me to find or do? What will the answer look like (e.g., a number of apples, a measurement, a comparison)?
  • Rephrase the question in your own words to ensure you understand the goal.

I – Identify a Strategy:

  • Based on your understanding of the situation and the question, decide what mathematical steps, operations, or procedures will help solve the problem (e.g., addition, subtraction, multiplication, division, drawing a diagram, making a table).
  • Consider if there are multiple steps needed. Use tools like graphic organizers or charts if they help plan your approach.

D – Do the Work:

  • Carry out your chosen strategy step by step.
  • Show your work clearly and write down your reasoning to track your thinking process and make it easier to review. Check calculations as you go.

E – Evaluate Your Answer:

  • Check your final answer: Does it make sense in the context of the situation you surveyed in step S? Is the unit appropriate for the question asked in step R?
  • Review your steps (D). If the answer seems unreasonable, revisit your understanding (S, T, R) or your strategy (I) and try again.

Revised Infographic for the STRIDE Model v2

Here’s a quick revision featuring the STRIDE Model v2. Be sure to review each step with a critical eye, and remember that I am challenged in both art and math. 🙂

3 comments
0 FacebookTwitterPinterestLinkedinEmail
MathScience

Embedding Interactive Lessons with Desmos and PhET in Quizizz

by Miguel Guhlin
written by Miguel Guhlin

Want to manipulate variables in a simulation so your students can answer questions? Blend Desmos and PhET in Quizizz to change how you make abstract concepts tangible. Let’s see how to do that.

Interactive Tools That Matter

When learning math, I often wondered how to get my hands on it. Absent manipulatives, you had to settle for understanding abstract concepts. For many students learning math and science concepts, not much has changed. They are still conceptual, ideas in one’s mind. That’s why interactive tools like those below bridge the gap:

  • Desmos graphing calculators
  • PhET simulations
  • Other virtual manipulatives

These tools allow students to make the intangible more than theoretical by:

  • Allowing students to manipulate variables,
  • Visualize relationships, and
  • Develop intuition through exploration

Now, those tools are available in Quizizz activities. This means students get those benefits and teachers get assessment data. Teachers need not switch between one platform to another. This facilitates just-in-time interventions.

Benefits of Desmos and PhET in Quizizz

Without having to switch from one platform to another, students can:

  • Explore concepts through hands-on digital manipulation
  • Immediately apply their observations to answer questions
  • Receive instant feedback on their understanding
  • Stay engaged in a single, cohesive learning experience

Let’s take a look at how to do that.

How to Embed Desmos in Quizizz

It’s not hard to embed Desmos calculators into Quizizz lessons. Here’s how to do it:

Approach #1: Embedding in a Quizizz Lesson

  1. Find or create your Desmos activity and copy the URL
  2. In Quizizz, edit or create a lesson
  3. Click “Add new slide” and select “Web page link”
  4. Paste your Desmos URL and save

Method 2: Creating a Passage with Questions (Recommended)

  1. Copy the web address (URL) from your Desmos activity
  2. In Quizizz, select “Create” and then “Passage”
  3. Click “Embed website” and paste your Desmos URL
  4. Add questions alongside the embedded calculator to check understanding
  5. Publish and assign to your class.

The second method offers significant advantages. It combines exploration with assessment. This gives the teacher immediate data on student understanding. It happens even as they they interact with the math tools.

Integrating PhET Simulations in Quizizz

The goal of PhET simulations is to bring scientific concepts to life. They achieve this through interactive, research-based simulations. Quizizz has partnered with PhET to make the integration of the two more seamless.

Screenshot of Quizizz search page for "phet" search term
Screenshot by author of Quizizz search screen

To use existing PhET simulations in Quizizz:

  1. Search for “phet” in the Quizizz library
  2. Browse the pre-made simulations with integrated questions
  3. Select one that fits your lesson goals
  4. Click “Start” to use immediately or “Assign” for later use

To customize or create your own PhET simulation activity:

  1. Find a pre-made PhET activity and click “Copy and edit”
  2. OR create from scratch by selecting “Create” → “Passage”
  3. Click “Embed website” and paste the URL from the PhET simulation
  4. Add your own questions tailored to your learning objectives
  5. Publish and assign to your students

Finding Pre-Made Interactive Resources

The Quizizz library already contains many activities with embedded Desmos calculators. As you saw in the screenshots above, it also has PhET simulations. To find them for use, follow the steps below:

  1. In the Quizizz search bar, type “Desmos” or “PhET”
  2. Use filters to narrow results by grade level and subject
  3. Preview activities to find ones aligned with your learning goals
  4. Use as-is or click “Copy and edit” to customize

For PhET, Quizizz collaborated with five science educators. They created a comprehensive library of simulations with aligned questions. These questions cover topics across physics, chemistry, biology, and earth science.

Ways to Use Interactive Quizizz Lessons

Want to get the best use of these embedded interactives?

  • Begin with clear learning objectives
  • Provide structured exploration guidance in your questions
  • Include a mix of conceptual and application questions
  • Use the 18+ question types in Quizizz to vary assessment approaches
  • Review class data to identify misconceptions for targeted follow-up

Take Your Interactive Lessons to the Next Level

Once you have the basics of embedding interactive tools, try these strategies:

  • Create a sequence of lessons that build conceptual understanding
  • Use different question types to target various learning modalities
  • Integrate open-ended questions that ask students to explain patterns they notice
  • Design collaborative activities where students predict, test, and explain using the simulations

A Quick Recap of the Benefits

Let’s review what makes embedding interactive lessons such a worthwhile effort:

  • You build student understanding through the activity
  • Students connect their explorations to the underlying concepts
  • Students get a more durable learning experience.

Start now with an embedded interactive lesson today. See how students can engage with concepts in a tangible, concrete way.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
Created by author
Content AreasEducational ResearchMath

Got GRITS? A Coaching Strategy for Lesson Study Groups

by Miguel Guhlin
written by Miguel Guhlin

Understanding fractions—especially adding and subtracting with unlike denominators—can be a major hurdle for fifth-grade students. Many struggle with conceptualizing the process beyond rote memorization. To address this, a structured lesson study approach can be highly effective. The GRITS Model (Goal Setting, Research, Implementation, Team Analysis, and Strengthening) provides a roadmap for refining instruction and ensuring deeper student comprehension.

Let’s explore how the GRITS model can be applied to improve student learning in this crucial math skill.

G – Goal Setting & Planning

“A well-planned lesson is the foundation of student success,” says Julia, a third-grade teacher.

Teachers choose a learning goal, identify student needs, and plan a lesson to address them. With a coach, teachers identify a learning goal. This goal should be based on student needs, curriculum standards, or instructional challenges. A great way to determine this? A low-stakes formative assessment, such as an entry ticket. With the data in hand, a coach and teacher can work together and put a lesson together.

Key Actions:

  • Anticipate potential student misconceptions and plan how to address them.
  • Review student performance data to identify gaps or misconceptions.
  • Align the lesson with curriculum standards and long-term learning objectives.
  • Set measurable outcomes for student understanding.
  • Determine the best instructional approach (e.g., hands-on learning, inquiry-based methods).

Objective:

Students will develop a strong conceptual understanding of adding and subtracting fractions with unlike denominators using visual models and real-world applications.

Lesson Plan Draft:

  • Utilize fraction bars and number lines to illustrate sums and differences.
  • Introduce a real-world scenario (e.g., sharing different-sized pizza slices).
  • Guide students in finding a common denominator and solving problems step by step.

By beginning with clear objectives and a well-structured plan, the team ensures alignment with student needs and curriculum standards.

R – Research & Lesson Development

“The best teaching is rooted in evidence and experience,” says Mark, an instructional coach.

Educators study best practices, research effective strategies, and refine the lesson plan based on evidence and expertise. Here are some key questions, research sources, and lesson adjustments to consider.

Key Actions:

  • Plan formative assessment checkpoints to gauge understanding during the lesson.
  • Consult educational research on effective teaching strategies.
  • Explore high-impact teaching strategies (e.g., scaffolding, peer teaching, manipulatives).
  • Consider different learning styles and differentiation strategies.
  • Develop lesson materials, including worksheets, visual aids, and real-world examples.


Key Questions to Guide Research:

  • What misconceptions do students typically have about fractions?
  • What strategies help deepen conceptual understanding?
  • How can we ensure students see the connection between visual models and algorithms?

Research Sources:

Lesson Adjustments Based on Research:

  • Incorporate collaborative problem-solving with manipulatives.
  • Add a self-reflection component where students explain their thinking.

Through research-driven adjustments, educators refine their approach to maximize student engagement and comprehension.

I – Implementation & Observation

“Seeing student reactions in real time helps us refine our approach,” says Lisa, a fifth-grade teacher.

One teacher teaches the lesson while others observe how students engage and respond. This portion is not unlike pineapple charts:

If you have a lesson planned that you believe other teachers might be interested in, whether it’s for a particular teaching technique you’ll be using, a new tool, or a powerful way to differentiate learning, you post that information in the faculty workroom (or anywhere teachers gather) on a pineapple chart. The chart is based on the idea of a pineapple as a symbol of welcome and includes all the days of the week and each period of the day. You post your interesting lesson on the chart on the specific day and time when you’ll be teaching it and thus invite your colleagues in to watch you in action. Here’s a sample.

You might consider combining these strategies. Let’s take a closer look.

Teaching Phase:
One teacher delivers the lesson while the rest observe student engagement. The lesson includes:

  1. Engage: Start with a relatable question (e.g., “If one person eats ⅓ of a pizza and another eats ¼, who ate more? How can we figure this out?”).
  2. Explore: Use fraction bars and number lines to model different sums.
  3. Explain: Guide students in finding a common denominator step-by-step.
  4. Elaborate: Provide real-world and word problems for practice.
  5. Evaluate: Have students explain their reasoning using visuals.

Observation Focus:

  • Are students using visual models effectively?
  • Are they able to explain why they need a common denominator?
  • What misconceptions arise?

During this phase, teachers gather insights on student understanding and instructional effectiveness. Some insights might be:

  • “Hands-on materials helped, but a few students played with the fraction bars instead of using them to solve problems.”
  • “A number line helped students compare fractions, but they had trouble placing mixed numbers correctly.”
  • “When students found a common denominator, they often forgot to adjust the numerators accordingly.”

What other insights can you imagine? Now that this phase is done, move to the next.

T – Team Analysis & Reflection

“Collaboration turns good lessons into great ones,” says Brian, a math specialist.

The group reviews student work, discusses observations, and identifies what worked and what needs improvement.
Post-Lesson Discussion:

  • What worked well? (e.g., students engaged with the pizza problem, strong participation in group work)
  • Where did students struggle? (e.g., some still tried to add numerators and denominators directly)
  • How can we improve the lesson? (e.g., provide more scaffolded examples before moving to word problems)

Student Work Review:

  • Examine student explanations and models.
  • Identify patterns in misconceptions.
  • Discuss targeted interventions.

This reflection stage ensures the lesson is continually refined to meet student needs. You might even try the PRISM Framework sentence stems to see how they work:

  • “I notice that many students describe [concept] as…”
  • “A common way students explain this idea is by saying…”
  • “The pattern I see in student models is that they often include/miss…”
  • “Here, I noticed that students frequently misunderstand…”
  • “A common error students make is assuming that…”

Give it a try yourself.

S – Strengthening & Sharing

“Our greatest impact comes from learning together and growing as educators,” says Sophia, a PLC facilitator.

The lesson is revised, possibly re-taught, and the insights are shared with other educators.
Lesson Revisions:

  • Add a warm-up activity where students estimate sums before solving.
  • Introduce color-coded fraction bars to differentiate denominators.
  • Provide more think-pair-share moments to solidify understanding.

Re-teaching: A different teacher implements the revised lesson while the group continues to observe and refine.

Sharing the Findings:

  • Document insights in a school-wide professional learning community (PLC).
  • Create a resource guide for other teachers on effective fraction strategies.
  • Consider making an instructional video for staff development.

The Power of the GRITS Model in Lesson Study

By following the GRITS cycle, coach and teachers create a dynamic, responsive approach to instruction. This model ensures that lessons evolve based on real student responses. Since it’s not based on assumptions or one-person’s perspective, it can make professional learning both practical and impactful. Whether applied to math or any other subject, the GRITS Model empowers teachers to get better at teaching, and coaches at coaching.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
Classroom ActivitiesContent AreasMath

Community Resource Roundup: Math Fact Fluency

by Macee Hall
written by Macee Hall

Ali Worsham, an Instructional Coach at Pine Tree ISD, recently asked the TCEA community, “What do y’all use for practicing math fact fluency? We currently use Xtra Math for our morning math drills, however a lot of our students have completed all of it. We have some using GimKit and paper based practices, and we chose not to renew our Reflex math subscription this year. We’re just looking for other options to keep it fresh!”

Well, that post got a ton of great answers that are jam packed with fun and engaging resources — so we’re sharing them with you! Check out some of our top community responses to that post below.

Math Fact Fluency Resources for Your Classroom

Karen Eden, a third grade math and science teacher at Georgetown ISD said…

“I have my students play games. I have played Pop-it multiplication with a 10×10 pop-it and students roll a dice (or two) to get their multiplication fact and then create the array on the pop-it. They also play Trash, which is a card game. I made 40 index cards with the multiples on them (so, 4 sets of multiples), and the kids lay the cards out face down in a ten frame. The first player picks a card from the draw pile and places that card in the correct spot where it would go in the ten frame, and then flips that card over and places it in the correct spot. Play continues like that until the first player gets a duplicate multiple, which then “goes in the trash”. The next player can then take that multiple out of the trash or pick a new card from the draw pile. The first player to reveal all 10 multiples wins that round. Circles and stars is also another great game to practice fact fluency and just requires a pair of dice. Students can fold their paper into 8ths and then draw the circles and stars in each box on their paper. I have students place a check or an x whether they win or lose the round (highest product wins). Games are great, fun ways to get students practicing their fact fluency!”

Jo Ellen Clive, a technology instructional coach at Scarborough Public School said…

“We are using Multiplication by Heart. They were bought out by Desmos recently, so the login is different than previous years, but it still works the same from the student end. Basically, it’s a daily 30-problem practice set of facts. It uses the concept of Spaced Repetition for frequent review and building mastery. The teacher can view student progress from their dashboard. In addition to multiplication, there are also practice decks for Number Recognition, Addition & Subtraction, and Division.”

Tiffany Crosson from Northside Christian School said…

“Boddle Math is free and you can assign addition/multiplication/etc. math facts for kids to practice.”

Sanna Elza, a third grade math and science teacher at Friendswood ISD, said…

“SplashLearn is what I use! It is free and has a gamified curriculum that is aligned to the TEKS! The kids love it!”

Dina Francisco, an instructional technology coach at the Memphis Business Academy, said…

“MobyMax is great for Math Facts, it starts with addition, then subtraction, multiplication, and then division. The nice thing about it, I really liked was the report you could print and send home to show progress.”

Katie Endorf, an educational technologist at Christ Community Lutheran School, said…

“Number Hive is another great math practice resource. No data tracking on the free version but it’s a very reasonably priced program. Works on any device, there is an app. Good math fact practice and they can compete against a classmate.”

Which resource is your favorite?

Thank you to everyone who contributed to this discussion in the TCEA Community! Which resource are you excited to use first? Let us know (and keep the suggestions coming!) in the comments below.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
robot
Math

Free Equatio Alternatives: Top Editors for Math Teachers

by Miguel Guhlin
written by Miguel Guhlin

Dear TCEA Responds:

Now that the free version of Equatio for educators is going away, what options for equation editors do you plan on using? My math teachers absolutely loved Equatio. I’m looking for some options that will make them equally happy. 🙂 Thank in advance for your help.
-Marcy

Dear Marcy,

Marcy, thanks so much for asking this question about Equatio, an equation editor that will no longer be free after June 30, 2024. Who wouldn’t be looking for tools to make creating and sharing mathematical content easier? In this blog entry, you will be introduced to four free alternatives to Equatio and a fifth not so free one. What’s more, you will get a LaTeX Reference Guide for Teachers to assist you.

Before you see those alternatives, let’s circle back and see what’s changed to make teachers consider other options for Equatio.

Equatio Update

As you point out, Marcy, Texthelp’s Free for Teachers program for Equatio came to an end on June 30, 2023. If you still had a current account under that program, you had until June 30, 2024 to find something else to use. At that point, access to Equatio is no longer free. Equatio DOES offer a 30-day free trial, however, if you want to test it out.

Why was Equatio so wonderful? Aside from the fact it was free, it offered these features:

  • Easily create math expressions including equations and formulas
  • Compatible with Google Docs, Forms, Slides, Sheets, and Drawings (Get the Chrome extension)
  • Input via keyboard, handwriting recognition (via touchscreen or touchpad) and voice dictation
  • Guesses what you’re typing or writing – like predictive text on your smartphone
  • Hear your math expressions read out loud
  • Compatible with LaTeX input for advanced users
  • Create interactive digital math quizzes
  • Input student responses directly into digital quizzes
  • Chemistry formula prediction

While Equatio was a popular choice when it was free, some may be looking to replace it now that it is no longer free. There are several alternatives available. Let’s explore five equation editors.

Option 1: LibreOffice Math

As an avid free Office suite user (see TCEA blog entries), LibreOffice has a lot to like. If you only use Google Workspace for Education or Microsoft Office, you may have missed out on a LibreOffice component. A part of the free LibreOffice suite, Math is a powerful formula editor that integrates seamlessly with other LibreOffice applications.

In the Classroom: Use Math to create worksheets with complex equations for high school algebra or calculus classes. For example, create a worksheet on solving quadratic equations, complete with step-by-step solutions.

Option 2: CodeCogs Equation Editor

Need an online editor that creates lightweight graphical equations? Check out CodeCogs. It generates code for easy embedding.

Some of its features (source) include:

  • Auto Complete – Instant suggestions for all supported mathematics LaTeX markup
  • Syntax Highlighting – Coloring of similar terms and underlining of matching elements
  • Fully Customisable – Change the arrangement, order, and content of any panel
  • Plugins – Compatible with major WYSIWYG Editor
  • fxRender – Fully integrated with the fxRender plugin for Microsoft Excel
  • Tables – Copy and paste tables from Excel and Word

What’s more, you can also embed it in a website. This means you can create a simple HTML page and then share it with your students.

In the Classroom: Middle school teachers can use CodeCogs to generate equations for online quizzes or digital worksheets. You might create a set of fraction addition problems and embed them directly into your class website or learning management system.

Option 3: Visual Math Editor

Visual Math Editor is a free, open-source LaTeX, AsciiMath, and MathML editor that works online or locally.

Some features:

  • PNG and SVG image exports
  • Works in all modern browsers without a plugin or extension
  • Includes more than 730 LaTeX symbols and 210 AsciiMath symbols
  • Available in multiple languages and colors
  • Offers a variety of editor equation samples

Explore the documentation online.

In the Classroom: You can use this tool to create visual math problems for learners or design colorful arithmetic equations with mixed operations for a math center activity.

Option 4: MathCast

MathCast is a free, open-source equation editor for Windows. You can input mathematical equations that are usable in documents, emails, and web pages. Around since 2004, it has a variety of features.

In the Classroom: High school geometry teachers can use MathCast to create detailed proofs with proper mathematical notation. For instance, develop a step-by-step proof of the Pythagorean theorem for a 10th-grade lesson.

MathCast Edit Screen via MathCast Help

Option 5: Symbolab

The last option available offers Google-based account creation and a free equation editor. Symbolab’s equation editor has step-by-step solutions that some will find helpful.That said, while there is a free version, you won’t get full access to all of its features. For about $40 a year, you have access to its entire feature set. See pricing online.

In the Classroom: Middle and high school algebra teachers can use Symbolab to create practice problems with detailed solutions for students to study. For instance, you can generate a set of linear equation problems with step-by-step explanations for an eighth-grade algebra class.

Some Parting Thoughts

By exploring these free alternatives to Equatio, you’ll be well-equipped to create professional-looking mathematical content for your lessons, assignments, and assessments without breaking the bank. Happy equation writing!

Handy LaTex Reference Guide for Teachers

Basic Operations

  • Addition: + → $a + b$
  • Subtraction: - → $a – b$
  • Multiplication: \times or \cdot → $a \times b$ or $a \cdot b$
  • Division: \div or / → $a \div b$ or $a / b$
  • Equals: = → $a = b$

Fractions and Decimals

  • Fractions: \frac{numerator}{denominator} → $\frac{a}{b}$
  • Mixed numbers: a \frac{b}{c} → $2\frac{1}{2}$
  • Decimal point: . → $3.14$

Exponents and Roots

  • Exponents: ^ → $x^2$
  • Subscripts: _ → $x_1$
  • Square root: \sqrt{} → $\sqrt{x}$
  • nth root: \sqrt[n]{} → $\sqrt[3]{x}$

Algebra

  • Variables: Just type them → $x$, $y$, $z$
  • Parentheses: (), [], or {} → $(x+y)$, $[a-b]$, ${1,2,3}$

Geometry

  • Angle: \angle → $\angle ABC$
  • Degree: ^{\circ} → $90^{\circ}$
  • Parallel: \parallel → $A \parallel B$
  • Perpendicular: \perp → $A \perp B$
  • Triangle: \triangle → $\triangle ABC$

Greek Letters (commonly used)

  • Pi: \pi → $\pi$
  • Theta: \theta → $\theta$
  • Delta: \Delta → $\Delta$

Calculus

  • Limit: \lim_{x \to a} → $\lim_{x \to a}$
  • Derivative: \frac{d}{dx} → $\frac{d}{dx}$
  • Integral: \int → $\int$

Sets and Logic

  • Element of: \in → $a \in A$
  • Subset: \subset → $A \subset B$
  • Union: \cup → $A \cup B$
  • Intersection: \cap → $A \cap B$
  • Therefore: \therefore → $\therefore$

Trigonometry

  • Sin: \sin → $\sin x$
  • Cos: \cos → $\cos x$
  • Tan: \tan → $\tan x$

Inequality Signs

  • Less than: < → $a < b$
  • Greater than: > → $a > b$
  • Less than or equal to: \leq → $a \leq b$
  • Greater than or equal to: \geq → $a \geq b$

A reminder: Enclose LaTeX expressions in dollar signs: $expression$ for inline math or $$expression$$ for displayed equations.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
Author generated image
Artificial IntelligenceEnglish Language Arts Reading (ELAR)MathScienceSocial Studies

New Free AI Tools from TeacherServer

by Miguel Guhlin
written by Miguel Guhlin

When I stumble across new educational technology tools, my heart races with excitement. It’s not about collecting amazing tools to fill my toolbox; it’s about finding those rare gems that can transform teaching and learning. Today, I’m sharing a few free tools from TeacherServer. Each of these has caught my eye and solved a real-life problem that teachers have. A bonus resource is included.

An screenshot by the author capturing a few of the tools available at TeacherServer.com.
An screenshot by the author capturing a few of the tools available at TeacherServer

Introducing TeacherServer

TeacherServer offers 47 different AI-powered tools, all available at no cost. A breakdown of these tools appears at the end of this blog entry. For now, here’s a list arranging the tools by grade level and function:

  • Elementary (K-5): six tools
  • Middle School (6-8): five tools
  • High School (9-12): five tools
  • All K-12: 16 tools
  • Adult Learners/Higher Education: four tools
  • Teacher Professional Development: 11 tools

TeacherServer’s collection of AI-powered tools is fantastic, and a great starting point. This is true even if you have not had much experience with AI.

Note: All images featured are screenshots, or generated, by the author.

How Do I Get Started?

You will need to create a free account with TeacherServer. Make sure you remember your password since it lacks an OAuth-type solution. That means you can’t log in with your Google Workspace or Microsoft 365 credentials.

Hot Tip: Use a free password management solution like Bitwarden or Keepass.

Get Hands-On: A Quick AI Tool PD Activity

Ready to jump into exploring TeacherServer? Grab a few friends before you read the rest of this blog entry.

Author generated image. Get a Copy link in text.
Author generated image. Get a Copy link in text.

Collaborate with a few colleagues to compare available tools on the TeacherServer. Consider using this note-taking document (View | Get copy of Canva template) to assist you in taking notes.

Activity Instructions:

  1. Form groups of three to four participants.
  2. Pick a category of tools from the TeacherServer list (see end of blog entry).
  3. Spend 20 minutes exploring the tools in your assigned category.
  4. Use the note-taking comparison chart provided in this blog to take notes on each tool you explore.
  5. After explore time elapses, each group will have five minutes to present their top two favorite tools.
  6. End with a ten-minute open discussion. Discuss how these tools would fit (or not) into daily teaching practices.

Discussion Questions

Some discussion questions to get you thinking:

  • Which tool surprised you the most and why?
  • How could these tools save you time in your daily teaching tasks?
  • What challenges might you face in implementing these tools in your classroom?
  • Are there any tools you wish existed that aren’t on the list?

Now that you’ve had a chance to explore these tools, here are a few of my favorites.

Tool #1: Tailor Your Texts with Precision

As a Language Arts teacher, finding text at the right lexile level can be a challenge. During an Elementary Technology Conference session, I relied on AI chatbots to create stories at grade level for Reciprocal Teaching practice.

The Lexile Score Adjuster on TeacherServer is a game-changer for differentiated instruction. This AI tool can generate a similar text at a higher or lower Lexile score. This makes providing reading materials matching each student’s reading level easier. You can simplify a complex text or challenge advanced readers with this tool and help you make sure that content you are teaching is reading accessible.

Tool #2: Spice Up Your Science Classes

Are you a science teacher? Give the Science Experiment Generator a try. It generates creative and safe science experiments for your classroom and takes the guesswork out of lab planning. You put in your grade level and topic and get a detailed experiment. This tool works for elementary to high school.

Here’s an example relying on grade 6 new science TEKS:

Tool #3: Master the 5E Model

No doubt, you have seen my other blog entries on the 5E Model. The 5E Model includes the following phases of learning:

  • Engage. Activities that capture the students’ attention, stimulate thinking. What’s more, they help students access prior knowledge, and focus on “how” or “why.” Phase of Learning: Surface Learning.
  • Explore. Enable students to explore ideas, alone or in groups. Provides time to think, plan, investigate, and organize information. Phase of Learning: Surface Learning.
  • Explain. Students get opportunities to connect their previous experiences. It also allows them to make conceptual sense of the main ideas of the topic under study. Phase of Learning: Deep Learning.
  • Elaborate. Students apply or extend previously introduced concepts and experiences to new situations. Phase of Learning: Transfer Learning.
  • Evaluate. Students with their teachers review and assess what they have learned and how they have learned it. It is a summative assessment. Phase of Learning: Transfer Learning.

TeacherServer will generate a 5E model lesson for you if you provide the grade level and topic, standard, or objective:

This tool helps you create daily, weekly, and unit lesson plans aligned with this popular teaching framework. It’s easy to miss the boat when it comes to addressing each element of the 5E model, but this tool can assist you in your planning.

Tool #4: Boost Your Students’ Vocabulary

If you are a K-3 teacher, you already know about the importance of a vocabulary program. It is a powerful high-effect instructional strategy. Vocabulary acquisition is the single most important factor in reading comprehension (source). TeacherServer has an amazing Vocabulary Builder that ggenerates exercises and activities to expand students’ vocabulary.

Like its other tools, you select your grade level and subject area. Then it provides you with a variety of engaging vocabulary-building activities. These can include any of the following:

  • Word game
  • Context-based exercise
  • Graphic organizer
  • Sentence completion

As an example, take a look at the sentence completion exercise below for a 12th grade math class:

After analyzing the data, we formed a _____ that the relationship between the variables was linear.

The graph of the function has an _______ at x = 2, which means the function approaches infinity as x approaches 2.

By ________the trend in the data, we can predict the stock market prices for the next quarter.

Based on the statistical analysis, we can ________ that there is a strong correlation between the two variables.

The graph of the quadratic function is a _______ that opens downward, indicating a maximum value at the vertex.

Another example includes creating a graphic organizer:

Create a graphic organizer with the following columns:

  • Vocabulary Term
  • Definition
  • Example Sentence
  • Context-Based Question

Sample directions for the activity include:

Ask students to complete the graphic organizer for each vocabulary term. Students should refer to the example sentences and context-based questions provided earlier. This activity helps students organize their thoughts and visualize the relationships between the vocabulary terms.

Author generated image from TeacherServer text using Mermaid code.
Author generated image from TeacherServer text using Mermaid code

Tool #5: Phonics and Reading Comprehension Coach

The Phonics and Reading Comprehension Coach creates phonics exercises and generates reading tasks for specific grades. This is helpful when you are targeting key literacy skills such as:

  • letter-sound relationships
  • Blending
  • Advanced reading comprehension strategies

Consider using it for the creation of differentiated reading assignments or to make intervention materials for struggling readers.

BONUS: Streamline Your Grading Process

The Assignment Feedback generator assists you in getting a second opinion for grading. You submit an assignment along with the rubric. Then you receive detailed feedback and comments. It may save you some time in grading. TeacherServer relies on a local AI server. This means you get super fast responses that don’t compromise privacy.

Wrapping Up

The best part of these tools? They’re completely free to use. Check back for new tools every week. TeacherServer has you covered, with all the activities below:

  • Adjusting text complexity
  • Planning science experiments
  • Structuring 5E lessons
  • Building vocabulary
  • Boosting phonics skills
  • Streamlining your grading process

Be sure to check out the many other tools they have available. Several charts appear below that highlight some ideas of how to best use them.

An Organized List of TeacherServer AI Tools

As nice as TeacherServer AI tools are, it can be a bit daunting to view a full-screen of what’s available. This list should narrow things down a bit for you. You may find a few tools repeated in different categories for your convenience.

Grade LevelTool NameDescription
Elementary (K-5)
Phonics and Reading Comprehension CoachCreate phonics exercises and reading tasks for specific grades.
Vocabulary BuilderGenerate exercises & activities to expand students’ vocabulary.
Daily Warm-Up Activity GeneratorCreate daily warm-up activities reviewing previous material.
Science Experiment GeneratorCreate creative and safe science experiments for the classroom.
Worksheet GeneratorGenerate a worksheet for your lessons on any topic and grade level.
Word of the Day (Weekly)Create a weekly poster that defines words, provides their meaning, and shows their usage in a sentence.
Middle School (6-8)
STEM Project DesignerGenerate ideas and plans for STEM projects to guide student inquiry.
Critical Thinking Exercise GeneratorDesign critical thinking exercises that challenge students.
Group Work Idea GeneratorCreate group work activities based on grade level and subject.
Mistake-Based Assignment CreatorGenerate assignments with intentional errors for students to identify and correct.
Quote of the Day ActivityCreate a quote of the day activity along with your grade level and subject area.
High School (9-12)
SAT Exam Question GeneratorProvide customized quizzes to enhance SAT readiness.
ACT Exam Question GeneratorCraft practice questions for the ACT exam.
Essay EvaluatorCritically assess student essays, highlighting strengths and areas for improvement.
Lexile Score AdjusterGenerate a similar text at a higher or lower Lexile score.
Text SummarizerCondense lengthy texts into concise, informative summaries.
All K-12
Lesson Plan GeneratorGenerate daily, weekly, and unit lesson plans aligned with curriculum goals.
5E Model Lesson Plan GeneratorCreate daily, weekly, and unit 5e model lesson plans aligned with goals.
K-12 Curriculum Quiz MakerDesign quizzes that align with the K-12 curriculum and learning objectives.
Formative Assessment GeneratorCreate various formative assessments to monitor understanding.
Assignment FeedbackSubmit your assignment along with the rubric to receive feedback.
Rubric GeneratorDesign clear and structured grading criteria for assignments.
Extracurricular ActivityPropose non-academic activities to foster holistic student development.
Classroom Case GeneratorProvide detailed classroom case studies to simulate challenges.
Student Behavior AnalysisEvaluate student behaviors to provide insights into classroom dynamics.
Behavior Intervention Plan GeneratorDetailed plan to help a student who is struggling to behave in class.
ESE Lesson ModificationsTailors lesson content and delivery methods to accommodate students with exceptionalities and special needs.
ESOL Lesson ModificationsAdapts instructional approaches to meet the unique requirements of English Language Learners.
Inclusive Accommodation PlannerDesign and customize accommodations for students, ensuring inclusivity and support for diverse learning needs in the classroom.
Generate a Classroom Climate SurveyDesign a classroom climate survey about students’ perceptions.
Text TranslatorTake any text and translate it into any language instantly.
Text ProofreaderCorrect grammar, spelling, punctuation, and add clarity to any text.
Adult Learners/ Higher Education
TOEFL Exam Question GeneratorGenerate targeted quizzes for TOEFL preparation.
Academic Blog Post GeneratorCraft engaging blog content on academic subjects.
Book Summary & ReviewEnter a book title to generate a summary and review.
PowerPoint Outline GeneratorDraft a structured outline for your PowerPoint presentations.
Teacher Professional Development
Professional Development PlanRoadmap for educators to enhance their skills and competencies.
Teacher CV CheckerReview and provide feedback on educators’ CVs.
Teacher Cover LetterGuide educators in crafting compelling cover letters.
Teaching Job InterviewPreparation tool with insights and practice questions for teaching job interviews.
Write Reference LetterDraft professional and persuasive reference letters.
FTCE Question GeneratorTailor practice quizzes to prepare for the Florida Teacher Certification.
FELE Question GeneratorTailor practice quizzes for the Florida Educational Leadership Examinations.
Peer Teaching EvaluationHighlight a peer faculty’s strengths and provide actionable improvement suggestions from teaching observations.
Lesson Style AssessmentAssess students’ learning styles and create tailored support strategies.
Classroom Case SolverUsers contribute classroom dilemmas while the AI provides solutions.
Write an Email in Multiple LanguagesGenerate a professional email and translate it into multiple languages.
Write a Multi-Language Email ResponseGenerate a professional email response and translate it into multiple languages.
2 comments
2 FacebookTwitterPinterestLinkedinEmail
OER
English Language Arts Reading (ELAR)MathScienceSocial Studies

The Essential Role of a Statewide Open Education Resource (OER) Repository

by Dr. Shannon Terry
written by Dr. Shannon Terry

In the pursuit of educational excellence, our nation is at a pivotal moment. While educators remain committed to providing every student opportunities to succeed amid competing priorities, resource restraints, and diverse perspectives, one state’s legislature is making significant strides by enhancing the availability and integration of OER that meets high-quality instructional materials (HQIM) criteria across classrooms in Texas. This major step forward refers to the Texas Open Education Resource (OER) Repository recently established under the state’s House Bill 1605. This centralized digital platform envisioned for Texas and designed to store, manage, and distribute educational resources and instructional materials is set to be a key driver in ensuring that high-quality, standards-aligned content is readily available to districts. The Texas OER Repository envisioned by legislators and representing learner-focused priorities of the state and districts is positioned to provide significant benefits for educators, students, and the broader educational system.

What Is a Learning Object Repository (LOR)?

A learning object repository is a digital collection of educational resources and instructional materials meticulously curated and easily integrated at the district level to support teaching and learning. These resources (also referred to as learning objects) are tagged with metadata (e.g., keywords, grade level, subject, and instructional standards) making them easily searchable and accessible to educators and students. As a centralized learning object repository that integrates seamlessly with various Learning Management Systems (LMS) and instructional tools, it catalyzes achieving educational goals and student outcomes by having high-quality, preferred resources available to classrooms just a click away. An example of a statewide learning object repository serving local districts is the Instruction Hub launched by the South Carolina Department of Education.

Key Features of an LOR for OER

  1. Centralized Resource Hub: A learning object repository houses all instructional materials in one place, ensuring that educators can easily find and use the resources they need. This centralization promotes consistency and quality across educational content.
  2. Standards Alignment: A learning object repository maps resources directly to state standards to allow educators to see which resources meet specific standards for each lesson. This further supports educators in understanding how each resource fits into the broader curriculum.
  3. Organizational Structure: A learning object repository supports the fidelity of implementation of HQIM by allowing resources to be accessed in the intended sequence. This facilitates the implementation of HQIM aligned to the designed learning path and state and district instructional frameworks.
  4. Seamless Integration: A learning object repository integrates with various Learning Management Systems (LMS) such as Canvas, Schoology, and Google Classroom, allowing teachers and students to access their specific resources effortlessly through Single Sign-On (SSO) and rostering capabilities.
  5. Customizable, Flexible, and Scalable: Districts and educators can add other HQIM resources to the learning object repository (e.g, other licensed content) allowing for customization to meet local needs. This flexibility ensures the LOR remains relevant and useful for diverse educational contexts. Plus, the LOR can be easily scaled and distributed making it an ideal solution for large and diverse educational systems like Texas.
  6. Data-Driven Insights: A learning object repository provides detailed analytics on how resources are used, enabling educators to measure engagement and the effectiveness of instructional content. These insights help refine and improve teaching strategies to better meet student needs.

The Benefits of a Learning Object Repository

The advantages of implementing a LOR are multifaceted, touching on efficiency, quality, and effectiveness in educational practices. Here are some key benefits:

  1. Time-Saving for Educators: Teachers spend a considerable amount of time searching for instructional materials. A learning object repository consolidates HQIM in one place, significantly reducing the time spent on resource discovery and allowing teachers to focus more on instruction and student engagement.
  2. Enhanced Teaching Quality: With access to a vast array of high-quality, standards-aligned resources, teachers can enhance their instructional practices. A learning object repository offers materials that are relevant, on grade level, and pedagogically sound, contributing to improved teaching and learning.
  3. Engaging Learning Experiences: A learning object repository enables the incorporation of different types of HQIM into lessons, including digital media, enhancing options for teachers to create engaging and interactive learning experiences for students. 
  4. Consistency Across Classrooms: One of the significant challenges in education is ensuring consistency in instructional quality across different classrooms and schools. A learning object repository promotes system-wide coherence by providing a centralized collection of vetted resources that all teachers can use to facilitate access by every student to a high-quality education.
  5. Bridging the Print-Digital Challenge: While by design a learning object repository manages resources digitally, it also supports materials designed for print delivery. This functionality ensures that students can benefit from HQIM regardless of their access to technology. Teachers can seamlessly transition between print and digital formats to provide a flexible and adaptable learning experience for all students.  
  6. Efficiency and Cost Savings: By reducing the need to purchase multiple instructional materials and eliminating redundancy, a repository can lead to significant cost savings for school districts. A learning object repository streamlines the process of updating and managing curriculum resources, ensuring that teachers always have access to the most current materials.

The implementation of a Texas OER Repository put forward by House Bill 1605 is a strategic move towards achieving educational excellence in the state. Its implementation promises to streamline access to HQIM, support effective teaching practices, and ultimately contribute to the academic success of every student in Texas. With Texas leading the way, this model will capture the attention of other states seeking to transform education and advance student success.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
Created by author, adapted from Mike Bell (2021) The Six Steps.
Artificial IntelligenceEducational ResearchEnglish Language Arts Reading (ELAR)HistoryMathReadingScienceSocial Studies

The LEARNS Cycle: Putting AI in Instruction, Part 1

by Miguel Guhlin
written by Miguel Guhlin

“There are a bucket-full of AI tools, apps, and suggestions, but how does any of this fit within the learning cycle? How does it align to evidence-driven, research-based instructional strategies?” Those are two questions that have been going around in my mind recently. It doesn’t matter if AI is the latest thing since sliced bread. How does it fit into teaching and learning?

To answer the question, I turned to the work of Mike Bell, author of The Fundamentals of Teaching. This blog entry makes an explicit connection between each step with AI tools available now to educators. Come along and see how AI fits what you already know works in the classroom. This is the first of a multi-part blog series that digs into this.

Finding Reliable Research and Strategies

Mike Bell’s work is genius in that it aligns the research of several different sources of evidence. In analyzing reliable sources of evidence, he overcomes several problems:

  • Confirmation bias taints our perception of evidence since people pick out evidence which supports their preconceptions.
  • Research can be contradictory, so it is necessary to combine results from different sources.
  • It’s hard to figure out the level of quality for research since not all research is of the same quality.

Since reliable evidence is hard to come up with on our own, all of us are tempted to listen to popular assertions. Bell suggests you do the following when someone (e.g. AI or an ed tech consultant or vendor) says, “The research says it’s effective:”

  • Ask for the effect size.
  • Gauge how much work is required to implement the suggestion.
  • Pause if the effect size is low and a lot of work is required to implement the suggestion.

A Quick Reminder about Effect Size

To that end, Bell offers an overview of effect size on learning, which readers of TCEA TechNotes will be familiar with:

  • 0: No effect
  • 0.2: Small effect
  • 0.4: Average effect (what John Hattie calls the “hinge point”)
  • 0.6: Good effect
  • 0.8 And above, high effect

Now that these key concepts are out of the way, let’s take a look at Bell’s Five Steps plus a post-assessment stage. These steps identify key strategies that are supported from several research sources. Those five major research studies include: EEF, Dean, Hattie, IAE, and IES. Explore these and other evidence-based research sources via this blog entry, Brain-Based Strategies That Work.

The Stages of the LEARNS Cycle

The five steps are detailed on Mike Bell’s website and include five big steps plus a post-assessment. The diagram below adapts the first five steps as The LEARNS Cycle. My goal was to place AI tool usage in the context of evidence-based teaching and see what might happen. Could AI tools be relied upon to assist with supporting classroom teaching and learning on a foundation of research-driven steps?

Created by author, adapted from Mike Bell (2021) The Six Steps.
Created by author, adapted from Mike Bell (2021) The Six Steps.

Here’s an overview of the LEARNS cycle:

LEARNS: Locate, Explore, Apply, Review, Nurture, Shine

  • Locate (Know what you know): Identify your current knowledge and skills related to the topic or learning objective.
  • Explore (Learn New Stuff): Discover and engage with new information, concepts, and resources to expand your knowledge and skills.
  • Apply (Challenge Yourself): Put your newly acquired knowledge and skills into practice by tackling challenging activities or real-world problems.
  • Review (Get feedback): Seek feedback from peers, mentors, or self-assessment to review your progress, identify strengths and weaknesses, and gather insights for improvement.
  • Nurture (Practice, Repeat, and Reflect): Continuously refine your knowledge and skills through repeated practice, reflection, and incorporation of feedback.
  • Shine (Re-assess, Progress Check, and Master): Re-assess your performance to track progress, ensure a high level of understanding, and achieve mastery of the subject matter.

What might putting Bell’s steps in our own words, and then connecting them to existing AI tools, look like? Let’s take a closer look at each of the stages in the recursive, iterative cycle. Remember, this is the first of a multi-part blog series.

1-Locate

In stage, you are activating prior knowledge. This involves knowing what you know, or what students know.

Locate (Know What You Know) Prior Knowledge

Instructional StrategyAI ToolDescription
Graphic OrganizersCreatelyCreately offers templates and drag-and-drop tools for Venn diagrams, concept maps, and flow charts, helping students organize information visually.
Structured Note-TakingNotion AINotion AI helps organize notes using methods like the Cornell method or mind mapping, making students’ notes clear and structured.
K-W-L ChartsPadletPadlet is a collaborative tool where students create and share K-W-L charts, activating prior knowledge and tracking learning progress.
Anticipation GuidesGoogle FormsGoogle Forms creates anticipation guides where students record initial thoughts and revisit them post-lesson to reflect on learning.
Case StudiesNearpodNearpod presents case studies and engages students in decision-making activities based on prior knowledge with the ability to revisit and adjust responses.

Here’s the infographic version:

A copy of the infographic will be available in the final blog entry in the series, so be sure to check back for the next part.

LEARNS Cycle: Sample Prompts

Another way to approach this is to generate prompts that supplement each strategy. These prompts would be usable in any AI chatbot, free or paid. This can be helpful if you do NOT have access to a paid solution, like MagicSolution.ai or the others I will mention in future blog entries.

Here are some sample prompts for the Locate stage. Note that the AI Tool Suggestions are tools you could use, but you could also just drop these prompts into a free chatbot.

Instructional StrategyAI Tool SuggestionsSample Prompt
Graphic OrganizersCreately, MindMeister, Lucidchart“Create a concept map to visually represent the key ideas and relationships in [topic].”
Structured Note-TakingNotion AI, Roam Research, Obsidian“Develop a structured note-taking system using the Cornell method to organize your understanding of [topic].”
K-W-L ChartsPadlet, Jamboard, Miro“Collaborate with your classmates to create a K-W-L chart for [topic], documenting what you already know, want to know, and have learned.”
Anticipation GuidesGoogle Forms, Microsoft Forms, SurveyMonkey“Complete this anticipation guide by indicating your initial thoughts on statements related to [topic] before we begin the lesson.”
Case StudiesNearpod, Pear Deck, Explain Everything“Analyze this case study on [real-world scenario] and make decisions based on your prior knowledge of [topic].”

This is what the K-W-L Chart prompt from above generates in assorted chatbots:

Author generated animated GIF composed of KWL charts generated by ChatGPT, Perplexity, MagicSchool, and Claude
Author generated animated GIF composed of KWL charts generated by ChatGPT, Perplexity, MagicSchool’s Raina, and Claude

Wait, There’s More AI Magic Available!

If you use school-focused AI tools, you may notice that there are none mentioned specifically in the chart for Locate stage in the cycle. You can find many of the activities for instructional strategies in these multi-purpose school AI tools.

AI Solution Providers: At the risk of incurring the ire of solution providers, here are what these look like in only one of the tools, MagicSchool.ai. In future blog entries in this series, I will introduce others.

Using MagicSchool.ai’s Raina, you can easily generate many of the items in this step. Let’s take a look at some sample prompts.

Screenshot of MagicSchool's Raina by author
Screenshot of MagicSchool’s Raina by author

Sample Prompts

Here are some example prompts (see 60+ prompt suggestions) that you can feed into any chatbot. These are meant to reflect actual tasks for the LOCATE stage of the LEARNS Cycle.

  1. Graphic Organizers (Science): “Create a graphic organizer for the seventh grade science unit on ecosystems. Use a concept map to show the relationships between producers, consumers, and decomposers, and how energy flows through a food web.”
  2. Structured Note-Taking (Social Studies): “For eighth grade social studies, organize notes on the American Revolution. Use the Cornell method to outline the causes, key figures, and major battles. Create a mind map to connect the outcomes of the war to the formation of the U.S. government.”
  3. K-W-L Charts (Science): “In sixth grade science, create a K-W-L chart for the unit on the water cycle. Begin by listing what students already know about evaporation, condensation, and precipitation. Include what they want to learn, and update the chart with new information as the unit progresses.”
  4. Anticipation Guides (Social Studies): “Design an anticipation guide for a fifth grade social studies lesson on the Civil War. Have students record their initial thoughts on the causes and key events of the war. After the lesson, revisit these thoughts and reflect on what they have learned and how their understanding has changed.”
  5. Case Studies (Science): “Present a case study for eighth grade science on the impact of human activities on climate change. Engage students in analyzing data on carbon emissions and their effects on global temperatures. Allow students to revisit their initial conclusions and adjust their responses as they learn more about the science behind climate change.”

ChatGPT Plus generated those sample prompts. Of course, you can write your own and then feed them into MagicSchool.ai’s Raina.

Getting Raina’s Help with LEARNS Cycle

In the screenshot above, you can see that Raina has taken the fifth grade social studies lesson prompt for an anticipation guide. The result is as follows:

Anticipation Guide for 5th Grade Social Studies Lesson on the Civil War

Before the Lesson:

Causes of the Civil War

I believe the Civil War was primarily about __.
I think the issue of states' rights played a significant role in starting the war. (True/False)
Slavery was a major cause of the Civil War. (True/False)
Key Events of the Civil War

The first battle of the Civil War was fought at __.
Abraham Lincoln issued the Emancipation Proclamation in the year __.
The Civil War ended at the Appomattox Court House in the year __.

After the Lesson:

Causes of the Civil War

My initial thoughts on the causes of the Civil War were __.
After learning more, I now understand that __ was a significant cause of the war.
My thoughts on the importance of states' rights in the Civil War have changed because __.

Key Events of the Civil War

Reflect on how your understanding of the first battle of the Civil War has evolved.
How did learning about the Emancipation Proclamation change your view of the Civil War?
Discuss how your perception of the end of the Civil War at the Appomattox Court House has shifted.
This anticipation guide will help students engage with the lesson content, reflect on their initial thoughts, and track their learning progression throughout the study of the Civil War.

Tying AI tools to proven effective instructional strategies is a non-brainer and will ensure that student learning is the focus.

0 comments
0 FacebookTwitterPinterestLinkedinEmail
Hand clicking on box to drag-and-drop it in the activity.
English Language Arts Reading (ELAR)ESL/ELLGood TeachingGoogle Tips and TricksHistoryMathScienceSocial StudiesWriting

Customizable Drag-and-Drop Google Slides Templates

by Dr. Bruce Ellis
written by Dr. Bruce Ellis

In today’s digital age, integrating technology into the classroom is not just an option, but a necessity. One effective way to do this is through interactive activities that engage students and make learning fun. One such activity is the drag-and-drop exercise, which can be easily created using Google Slides.

Google Slides, a versatile and user-friendly tool, is typically thought of as just a presentation tool. Since it is so easy to use however, it allows educators to design a variety of interactive activities that cater to different learning styles and needs. These activities can range from matching vocabulary words to definitions, sequencing events, categorizing items, solving math problems, and labeling diagrams. The possibilities are endless!

Using drag-and-drop activities in your teaching arsenal offers several benefits. They are engaging and interactive, encouraging students to actively participate in the learning process. They also provide a unique way to assess student understanding, offering immediate feedback and opportunities for differentiated instruction.

Below we explore the value of using Google Slides for creating drag-and-drop activities, the importance of varying your assessment strategies, and share some customizable templates that you can use in your classroom. Whether you are a tech-savvy teacher or just getting started with digital tools, you’ll find that Google Slides is a powerful ally in creating a dynamic and inclusive learning environment.

Value of Using Google Slides

Google Slides is an intuitive and user-friendly tool that doesn’t require extensive technical skills. Both teachers and students can quickly learn to create and interact with drag-and-drop activities. The simple drag-and-drop interface makes it easy to move elements around, fostering a hands-on learning experience.

And because Google Slides is accessible from any device with an internet connection, including computers, tablets, and smartphones, students aren’t limited to just using a shared classroom laptop. This accessibility ensures that students can participate in activities whether they are in the classroom or learning remotely. Additionally, Google Slides offers various accessibility features, such as screen reader support and keyboard shortcuts, making it inclusive for all students.

Value of Drag-and-Drop Activities

Drag-and-drop activities are highly engaging and interactive, capturing students’ attention and encouraging active participation. They cater to kinesthetic learners by incorporating physical movement, which can enhance understanding and retention. These activities also offer flexibility for differentiation, allowing teachers to tailor tasks to varying levels of difficulty and meet diverse student needs. By providing immediate feedback, drag-and-drop exercises help students quickly identify and correct mistakes, reinforcing learning in real-time.

Consider scaffolding so that students observe completed diagrams/models before moving to drag-and-drop activities. Afterwards, you can have students draw and label the diagrams themselves without the needed supports provided in drag-and-drop activities. 

In addition to these benefits, drag-and-drop interactives foster critical thinking and problem-solving skills as students analyze information and make decisions to complete tasks. They promote collaboration when used in group settings, encouraging teamwork and peer learning. These activities also break up the monotony of traditional instruction, making learning more dynamic and enjoyable. By integrating these tasks, educators can create a more engaging and effective learning environment that supports skill development and enhances overall student achievement.

Varying assessment strategies in the classroom is essential to accommodate the diverse learning styles and abilities of students. Traditional tests and quizzes may not accurately reflect every student’s understanding or potential. By incorporating a range of assessment methods—such as formative assessments, project-based learning, interactive activities, and peer evaluations—teachers can gain a more comprehensive view of student progress. Diverse assessments also keep students engaged and motivated, as they provide multiple ways to demonstrate knowledge and skills. This approach fosters a more inclusive and equitable learning environment, ensuring that all students have the opportunity to succeed and that instruction can be tailored to address their unique needs.

Looking for Drag-and-Drop Inspiration

When creating drag-and-drop activities, teachers can draw inspiration from various sources like game boards and graphic organizers. Game boards, with their clear and structured layouts, can be easily adapted into engaging educational activities. Consider classic board games such as Scrabble or Memory Match, where students can drag letters to form words or match pairs to reinforce vocabulary. Similarly, graphic organizers like Venn diagrams, flowcharts, and T-charts are excellent for visually organizing content, helping students categorize and sequence information. For instance, you can design a Venn diagram activity where students drag items into the appropriate circles to compare and contrast concepts. By examining how these tools organize information, you can replicate similar structures in your drag-and-drop activities, making them visually appealing and effective for student understanding and retention. So, don’t hesitate to draw inspiration from these familiar formats to create dynamic and interactive learning experiences for your students. A few sites to consider exploring include The Teacher Toolkit, HMH Free Graphic Organizer Templates, Canva Graphic Organizer Templates, and just searching for graphic organizer images. 

If your slide is going to have a diagram, such as parts of an animal cell, life cycle of a butterfly, or other instance in which students will drag-and-drop words to designate part of an image, consider searching images for ideas. Begin by searching for your term (i.e.: parts of an animal cell). If you are using Chrome, click on Images and then the Tools button. Next click on Color and choose Black and White. Here you should find many ideas of how to organize your drag-and-drop slide to accommodate the learning activity. Here are sample search results for parts of an animal cell, life cycle of a butterfly, parts of a flower, regions of Texas, and the chemical structure for glucose..

Steps to Creating Drag-and-Drop Activities

Creating drag-and-drop activities in Google Slides is straightforward and can be a fun way to engage your students. Here’s a step-by-step guide to help you get started:

Step 1: Set Up Your Google Slide

Setting up your Google Slide is quick and easy. If you plan ahead, you can reuse the slide for many different drag-and-drop activities.

  1. Open Google Slides and start a new presentation. Or, just go to http://slides.new. 
  2. Choose a blank slide to have a clean canvas to work on.
  3. Title your slide to indicate the type of activity (e.g., “Vocabulary Matching Activity”).
  4. Consider adding related graphics and images to the title slide. Use sites such as Pixabay and Pexels if you need free images. Or, if you have a Canva for Education account, search for related images within their repository.

Step 2: Design Your Activity Background

Create a visually appealing and organized layout for your activity. You can use shapes, text boxes, and lines to guide where students will place the draggable items.

  1. Go to the “Background” option in the toolbar and select “Choose image” to add a background image if needed. This can be a grid, a diagram, or any relevant image.
  2. You can also use shapes, text boxes, and lines to design the background directly on the slide. For example, create boxes where students will drag and drop items.
  3. In step 5 below you’ll make it so these items are one static image and cannot be moved or rearranged. 

Step 3: Add the Drag-and-Drop Items

Incorporate the elements that students will interact with, such as text boxes or images. Make sure these items are clearly labeled and easy to move.

  1. Insert the objects that students will drag and drop. These can be text boxes, images, shapes, or a combination.
  2. To add text, click on the “Text box” tool, click on the slide where you want to add text, and type in your content.
  3. To add images or shapes, use the “Insert” menu and choose the appropriate option. Resize and position these items as needed.
  4. You may want to group each text box on a shape or set the background of each text box so it stands out.

Step 4: Make Objects Movable

Ensure the draggable objects are separate from the background so they can be easily manipulated. This setup allows for a smooth drag-and-drop experience.

  1. Select the objects you want to be draggable by clicking on them.
  2. Ensure these objects are not part of the background. They should be separate elements that can be moved around on the slide.
  3. If there is not enough space on the slide itself, consider moving draggable words/content off to the side of the slide. 

Step 5: Lock the Background Elements

To keep your background intact, convert it into a static image. This prevents students from accidentally moving parts of the layout.

  1. To prevent background elements from being moved accidentally, create the background layout in a separate slide.
  2. Download this slide as an image (File > Download > PNG or JPEG).
  3. Create a new blank slide and set the downloaded image as the background (Background > Choose image).
  4. Alternatively, you can create the background elements using the Theme Building (View → Theme Builder), This would still allow you to edit the slide at a later date. When students are viewing the slide, though, items would appear to be locked.

Step 6: Provide Instructions

Include clear and concise instructions to guide students through the activity. Good directions help students understand the task and reduce confusion.

  1. Add a text box at the top or side of the slide with clear instructions for the activity. For example, “Drag each word to its correct definition.”
  2. Use concise and straightforward language to ensure students understand the task.

Step 7: Share with Students

Distribute the Google Slides presentation to your students, making sure they have the appropriate permissions to interact with the activity. Using platforms like Google Classroom can streamline this process.

  1. Once your activity is ready, share the Google Slides presentation with your students.
  2. Make sure to change the sharing settings to “Anyone with the link can edit” if you want them to interact directly with the slide.
  3. Alternatively, you can assign the slide via Google Classroom or another learning management system.

Step 8: Practice and Adjust

Test the activity yourself to identify any issues and refine it based on your findings. Student feedback can also provide valuable insights for improvement.

  1. Before using the activity with your students, test it yourself to ensure everything works as expected.
  2. Be prepared to make adjustments based on your observations or student feedback to improve the activity.

Drag-and-Drop Activity Templates for You

To help you get started, we’ve created a collection of customizable drag-and-drop activity templates that you can easily adapt for your classroom. These templates include a variety of activities such as vocabulary matching, sequencing events, categorizing items, solving math problems, and labeling diagrams. Simply copy the template you need, tweak it to fit your specific lesson, and you’re ready to engage your students with interactive and meaningful learning experiences.You can access the editable Google Slides template here.

screenshot of slides in drag-and-drop templates slide deck

Here is a drag-and-drop activity that has been created for fifthth grade social studies. Additional slides in the Theme Builder have been deleted while others have been added that are relevant to this activity. In this activity, students are expected to become secret agents to correct a timeline that has been messed up by a historical villain. After researching to correct the mistake, they work to find out other important information to complete the slide deck. When done, they present their findings to their team to successfully correct the error in history. The main image (excluding the words) was created in Ideogram (log in in see the actual image and prompt).

Drag-and-drop activities can be used for most content areas and in many grade levels. How you tweak it for second graders will differ from using drag-and-drop with high school students. And, as you include it as part of an assignment (as opposed to the complete assignment), students will find it to be a helpful learning aid as they solidify their understanding of the content they are studying.

Do you already use drag-and-drop activities in your classroom? If so, please share with us and let others know how you set your activities up and what students like most about them!

1 comment
0 FacebookTwitterPinterestLinkedinEmail
An 81 piece Quaker friendship quilt pictured on the left. On the right is a picture of one of the quilt blocks showing a hand-written message and signature.
HistoryMathSocial-Emotional Learning

Friendship Quilts Bring History, Math, and SEL Together

by Emily Horn
written by Emily Horn

You’ve heard of friendship bracelets… but what about a friendship quilt? Whether it’s for a back-to-school community builder, a social studies tie-in, or just a feel-good class project, creating a friendship quilt is a meaningful way to celebrate connection and belonging. First, we’ll explore the history and symbolism behind quilts—especially friendship quilts—and share a hands-on activity that brings students together, stitch by stitch (no sewing required). Bonus: You’ll get plenty of additional resources for weaving quilts into your lessons, too.

If you’d like to skip right to the project, click here.

Why Are Quilts Important?

My grandmother, Ma, made each of her children and grandchildren several quilts throughout our lives— Christmas quilts, quilts for graduation, quilts made of old blue jeans, quilts made from material with patterns representing hobbies or interests, etc. She wasn’t a show quilter or professional, but she would spend hours choosing material, measuring, piecing patterns, pinning, and sewing. My ever-patient grandfather, Pa, was called upon for all the meticulous cutting that my grandmother did not particularly enjoy. She would always include a little cross-stitched patch with our name, “Love, Ma,” and the date, which is my favorite part. I will cherish and keep these quilts forever!

Photo of a quilt with a cross-sticked message from the quilter.
Quilt made by Ma in 2002

As a young girl, my mom saved up all her extra money over time to get me an American Girl Doll, the absolute prized possession of so many children in the late 80s and 90s. I had wanted one for a long time! And my first experience with quilting myself was when my Ma helped me sew a small quilt to cover my American Girl Doll with at night, you know, while she was sleeping. I’ll never forget sitting with her, talking, and learning about sewing and how to cut and measure the squares. Now, this is just a bit of my personal experience with quilts and quilting, but it is a very teeny tiny reflection of how quilting is passed down and how relational it can be.

A 16x16 inch (4x4 quilt block) doll quilt sewn by the author and her grandmother.
16″ x 16″ babydoll quilt made with my Ma circa 1990.

Quilting is a skilled art passed from generation to generation. It spans cultures and peoples. Quilts require mathematical skills, creativity, patience, focus, and precision. They were, and are still, handmade and given to commemorate special occasions— a form of both artistic expression and documentation. Additionally, quilting bees and circles were a way for women to socialize and spend time together. It was at one time a very community-driven and relational art, and in some areas and cultures, this is still very much the case. Over time, there have been many different types of quilts and quilt patterns, like Double Wedding Ring, Patchwork, Log Cabin, Crazy Quilts, and more, but one of my absolute favorites is the friendship quilt.

1974 Friendship Quilt Displayed at The Airing of the Quilts, Greenwood, Arkansas
1974 Friendship Quilt | The Airing of the Quilts, Greenwood, Arkansas | “Made by Milltown Methodist Church and given to Rev. J.M. and Jane Rogers, Owned by Jane Rogers”
Note the signatures of the ladies who made each square on the white diagonal pieces.

What Is a Friendship Quilt?

Friendship quilts are a traditional form of quilt made collaboratively by a group of friends or even family. They have a rich history and special significance in American culture and are some of the very few quilts that have names associated with them. They sometimes even included dates, special messages, and addresses as well, making them very special. Originating in the Philadelphia, Baltimore, and New Jersey areas, the tradition of friendship quilts spread to become more popular in areas west, north, and south. The form and patterns of friendship quilts have changed and adapted over time.

The Making of a Friendship Quilt

A friendship quilt is made by having each member of a friendship group contribute a quilt block that they have sewn. The quilt blocks are then assembled and sewn together into a single quilt. Each block is unique and represents the individual who made it. The blocks often contain personalized decorative elements like embroidered names, initials, symbols, dates, or short messages. Names, initials, and messages may be hand-sewn, written in ink, or even stamped.

An 81 piece Quaker friendship quilt pictured on the left. On the right is a picture of one of the quilt blocks showing a hand-written message and signature.
Conner Prairie, Public domain, via Wikimedia Commons | 81 Piece Sampler Quilt (left) | Quilt Square (right) |
Quaker Album or Friendship quilt made in Burlington County, New Jersey in 1843.

The Meaning of the Friendship Quilt

Friendship quilts first became popular in the early 1800s. Groups of women would gather together for quilting bees as a social occasion. They would work collaboratively on quilts for members of their community while also using it as a chance to talk and build friendships. The quilt they produced represented the bonds of sisterhood, community, and connection between those who worked on it.

The History of the Friendship Quilt

These quilts were especially meaningful on the American frontier. As women headed west from the east coast with their families, the quilts served as a record of the long journey. They also helped pioneer women maintain social networks in isolated areas without extended family nearby. The quilts connected them to friends and home.

Over time, friendship quilts began to mark major life events like births, deaths, weddings, or relocations. They took on special significance as historical records of women’s lives and friendships. The communal creative process represents shared experience and cooperation.

1933 Carolina Lily friendship quilt displayed at The Airing of the Quilts in Greenwood, Arkansas.
Carolina Lily Friendship Quilt Displayed at The Airing of the Quilts, Greenwood, Arkansas | The quilt top was pieced by friends in 1933. In 2017, relatives and friends of the original group quilted it.

Today, many antique friendship quilts are preserved in museums. They are valued as culturally important folk art celebrating relationships between women and documenting American history through intricate stitches and patches.

Making a Class Friendship Quilt

If you’re looking for a class project, why not piece together a paper friendship quilt with students that represents your classroom community? Each student can make a quilt block to contribute to the class friendship quilt and sign their name on it. A quilt block is a unit (in this activity it will be a square) that is sewn together to make up a full quilt.

For this activity, students will each construct their own quilt blocks out of paper. Essentially, they will:

  1. Cut the shapes out of a quilt block pattern.
  2. Trace the shapes onto a variety of colorful, patterned paper.
  3. Cut those colorful shapes out.
  4. Glue them onto a plain white square made of posterboard, card stock, or paper.
  5. Admire their created quilt blocks!

Here is what a finished quilt block made by a student might look like. This was a student-created pattern, meaning the student did not follow a quilt block pattern but made up their own using shapes.

Example Paper Quilt Block

Here is a list of materials and a few suggestions for choosing quilt block patterns. It can really be as simple or complex as you’d like it to be!

Materials List:

Three examples of quilt block patters that could be used for a friendship quilt.
Screenshot by the author: Example Quilt Block Patterns from Free Quilt
  • Plain white square (6×6, 9×9, or 12×12) made of posterboard, cardstock, or paper.
  • Quilt Block Patterns: When it comes to patterns, I’ve listed a few approaches for you to choose from. Choose which option works best for you and your students.
    • Pattern Option 1: Preselect one quilt block pattern for all students to use as their template. Distribute a copy of the pattern to each student in the correct size.
    • Pattern Option 2: Preselect 3-4 quilt block patterns for students to choose from for their template. Distribute a copy of the pattern to each student in the correct size.
    • Freeform Option: Have students create their own special patterns using traceable shapes, rulers, or freeform drawing!
    • Digital Option: Have students design their blocks online using line tools, shapes, and elements in Google Slides, Canva, Adobe Express, or similar tools.
  • Paper: Scrapbook paper, wrapping paper, and colorful paper with a pattern will work great. Some solid paper would also be good to incorporate. Note: For younger students, sticking with just solids may be best if you’re having them follow a quilt block pattern.
  • Crayons, Markers, Colored Pencils: If you opt for students to create their own patterns on white paper, you’ll need plenty of coloring options!
  • Scissors: Regular scissors or scissors with fun edges are both great.
  • Glue: You can use glue sticks, from a bottle, or Mod Podge (I prefer using old paint brushes with liquid glue).
  • Ink: Sharpies or paint/gel pens are great.
  • Ruler: Measuring to make sure the squares and pieces are straight and the correct size will be important!
  • Pencil: Mistakes will happen. Using a pencil when measuring and tracing will be helpful.

This can be just a fun arts and crafts activity for students to celebrate Palentine’s (or Valentine’s) Day. But you can also incorporate some math and history standards as well!

Suggested Lesson Plan Structure

This is an adaptable lesson that can be completed in a short period or span multiple periods. Feel free to adapt it to meet your needs and desired outcomes! For example, if you’d like the activity to go more quickly, you can provide precut shapes for students to glue (no cutting or tracing involved) or have them design squares in Canva to be printed. If you’d like to take more time, incorporate some of the math ideas and history of friendship quilts.

Screenshot by the author of a Quilt Block Template Being Created in Canva
Screenshot by the author: Quilt Block Template Being Created in Canva

Introduction:

Introduce students to the concept and history of the friendship quilt. Look at pictures of different friendship quilts and talk about what students notice. How do students imagine these quilts were made? What do friendship quilts tell us? Why might they be important in a community or group of people? Read an article or book as a class and discuss (see resource list). Explain that students will be making a paper friendship quilt as a class. Each student will contribute one square, and when they are all put together, it will create a full-sized paper quilt.

Activity:

Explain and briefly demonstrate how:

  • Students will either choose a block pattern or create their own block pattern for their square. Look at some examples of quilt block patterns together, if appropriate.
  • Explain and demonstrate how students will cut out the shapes from their printed block pattern and trace them onto the colorful paper of their choice.
  • Then, show students how to cut the traced shapes from their colorful paper and position them on their white squares. They should lay out their shapes to match their block pattern.
  • Demonstrate how to glue the pieces of the block to the plain white square.
  • Show students an example of a finished square. You could make your square in advance to show them!
  • Distribute the quilt block pattern you chose, have students choose a pattern, or let them create their own patterns.
  • Pass out the plain white squares (whatever size you choose, 6″, 9″, 12,” etc.).
  • Have fun cutting, tracing, gluing, and signing squares!
  • Hang students’ blocks individually on the wall, grouping them together to create a visual quilt. Or create your quilt by gluing the squares together before hanging the full quilt on the wall.

Ideas for Incorporating Math Skills

After you determine how big you would like your overall quilt to be, here are some math skills you can work with students on before and during the activity:  

  • Determine the perimeter and area of the full quilt.
  • How many rows and columns should the quilt have in order for each student to have the same size square? Calculate how big each individual student’s square should be.
  • Identify the shapes within your block. 
  • Explore symmetry by creating block patterns with symmetrical sides.
  • Measure and record the angles of the shapes within your block. 
  • If designing your own pattern, use a ruler to draw out and measure shapes in your pattern. Record your measurements.

Lesson Plans and Resources about Quilts

Besides friendship quilts, there are many other varieties of quilts, each with their own significance and meaning. I hope you’ll enjoy exploring some stories, history, resources, and information on the great personal and historical significance quilts hold. And if you try making a friendship quilt with your students, please leave us a comment and link to a photo! We’d love to see your class’s friendship quilt.

0 comments
1 FacebookTwitterPinterestLinkedinEmail
Newer Posts
Older Posts