“Cross multiply… just do it! Don’t ask why! It works every time!” This was an unspoken rule when I learned fractions years ago. Many of us had the same experience. Our fraction sense was a series of memorized steps. Where did it get us? It made us human calculators who lack the ability to reason about the size of fractions.
With “new math,” students have skills that build strong conceptual knowledge using strategies that build number sense such as decomposition. Decompose means to break down into parts. Students usually perform well at this skill. They are able to decompose whole numbers such as 10 is 2 + 8 or 10 is 2 + 3 + 5. This level of thinking can be used when reasoning about fractions. Let’s use what the kids know to help them make connections to concepts they do not know.
This starts with the way we explain the meaning of a fraction. The numerator is more than the top number that tells us how many are shaded or not shaded. The denominator is more than the bottom number that tells us the number of objects in all. Many times our students interpret these definitions to mean that there are two whole numbers, one on the top and one on the bottom. They see the fraction as two separate whole numbers rather than a number that has a value.
Let’s modify the way we define the parts of a fraction to students. According to John Van de Walle in “Elementary and Middle School Mathematics, Teaching Developmentally,”:
“the numerator counts and the denominator tells what is counted”.
These definitions are aligned to the level of thinking students should be encouraged to use as they build number sense as it relates to fractions.
- Let’s look at an example, 3/8. In this fraction, the denominator is 8. So, we know we are counting eighths. The numerator is 3, which tells us we are counting 3 one-eighths.
When students use this information to decompose fractions into their parts, they are able to reason about the size of a fraction. They should be able tell you several truths about the fraction 3/8. Here are a few examples that have been modeled using the online tool Mathigon.org. It is an awesome website that can be useful to help students explore and make sense of fractions.
- The whole is 8 eighths or 8/8.
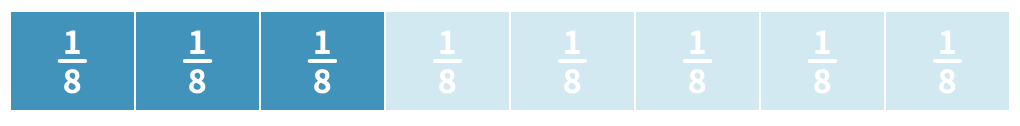
- 3/8 can be renamed to 1/8 + 1/8 + 1/8. These are three iterations of copies of 1/8.
- 1/2 of 8/8 is 4/8.

- 4/8 = 1/2 So, 3/8 < 1/2 or 1/2 > 3/8.
Conclusion
Decomposing is a powerful tool in developing number sense. When students know the meaning of a fraction, they are able to determine the whole, the unit fraction, and reason about sizes. We must take advantage of this and ensure we allow students to explore and justify their reasoning while using the correct language. Sometimes this takes a shift in our thinking, but we have to ensure we are meeting the kids where they are instructionally and not expecting the kids to meet us where we are. The kids can do it when we help them make the connections.

